Articles
- Page Path
- HOME > Restor Dent Endod > Volume 32(1); 2007 > Article
- Original Article The influence of composite resin restoration on the stress distribution of notch shaped noncarious cervical lesion; A three dimensional finite element analysis study
- Chae-Kyung Lee1, Jeong-Kil Park1, Hyeon-Cheol Kim1, Sung-Gwan Woo2, Kwang-Hoon Kim2, Kwon Son2, Bock Hur1
-
2007;32(1):-79.
DOI: https://doi.org/10.5395/JKACD.2007.32.1.069
Published online: January 31, 2007
1Department of Conservative dentistry, College of Dentistry, Pusan National University, Korea.
2Department of Mechanical Design Engineering, College of Engineering, Pusan National University, Korea.
- Corresponding Author: Bock Hur. Department of Conservative Dentistry, College of Dentistry, Pusan National University, 1-10, Ami-dong, Seo-gu, Busan, Korea, 602-739. Tel: 82-51-249-7455, bhur@pusan.ac.kr
Copyright © 2007 Korean Academy of Conservative Dentistry
- 169 Views
- 2 Download
- 1 Crossref
Abstract
-
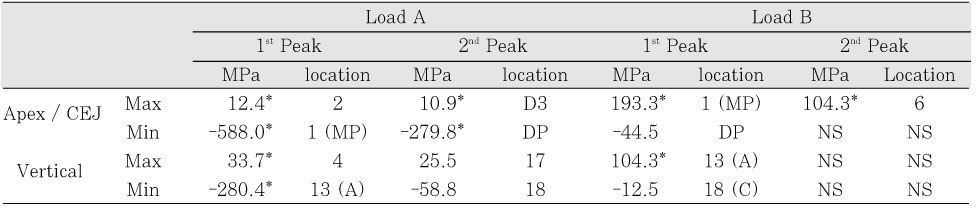
The purpose of this study was to investigate the effects of composite resin restorations on the stress distribution of notch shaped noncarious cervical lesion using three-dimensional (3D) finite element analysis (FEA).Extracted maxillary second premolar was scanned serially with Micro-CT (SkyScan1072; SkyScan, Aartselaar, Belgium). The 3D images were processed by 3D-DOCTOR (Able Software Co., Lexington, MA, USA). ANSYS (Swanson Analysis Systems, Inc., Houston, USA) was used to mesh and analyze 3D FE model. Notch shaped cavity was filled with hybrid or flowable resin and each restoration was simulated with adhesive layer thickness (40 µM). A static load of 500 N was applied on a point load condition at buccal cusp (loading A) and palatal cusp (loading B). The principal stresses in the lesion apex (internal line angle of cavity) and middle vertical wall were analyzed using ANSYS.The results were as follows1. Under loading A, compressive stress is created in the unrestored and restored cavity. Under loading B, tensile stress is created. And the peak stress concentration is seen at near mesial corner of the cavity under each load condition.2. Compared to the unrestored cavity, the principal stresses at the cemeto-enamel junction (CEJ) and internal line angle of the cavity were more reduced in the restored cavity on both load conditions.3. In teeth restored with hybrid composite, the principal stresses at the CEJ and internal line angle of the cavity were more reduced than flowable resin.
I. Introduction
II. Materials and methods
Loading conditions
Restorative materials and adhesive layer thickness
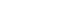
 Figure 3
Figure 3 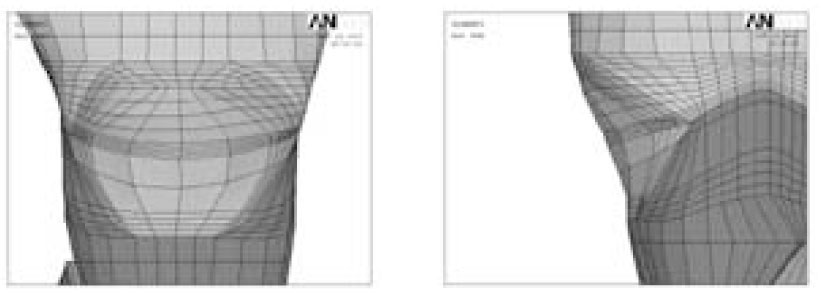 Figure 2
Figure 2
Groups for simulation
Stress analysis
III. Results
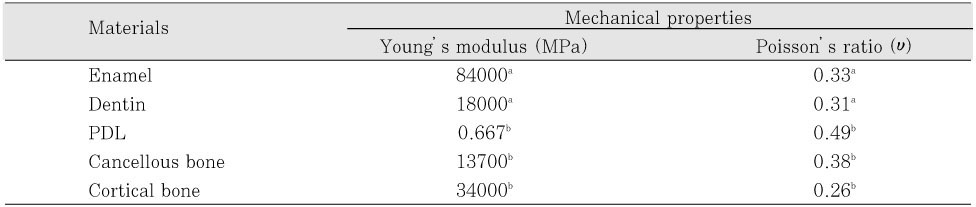
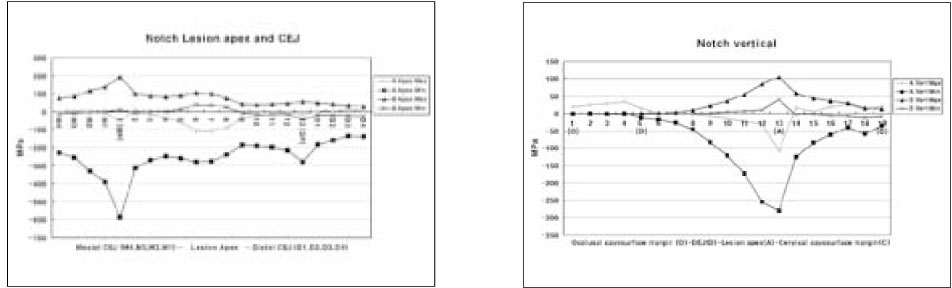 Figure 4
Figure 4 *: Excessive stresses over the failure range
A: Lesion apex, C: Cervical cavosurface margin, MP: Mesial point angle, DP: Distal point angle, NS: not significant
 Download Table
Download Table 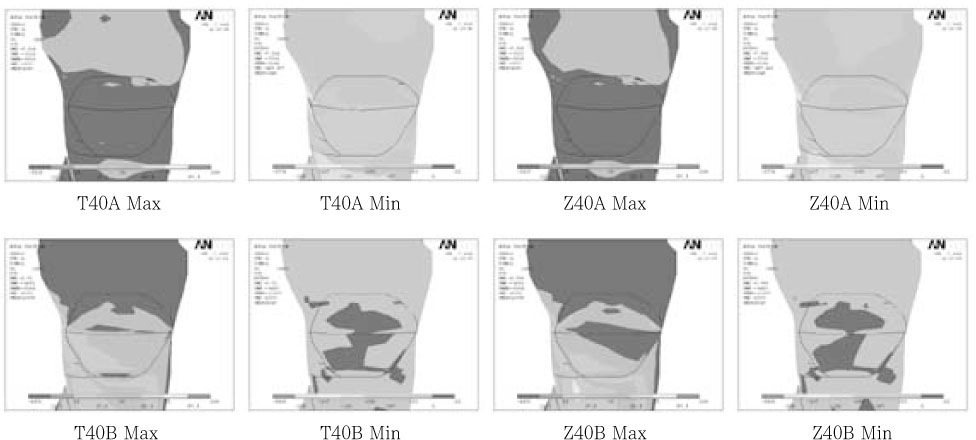 Figure 5
Figure 5 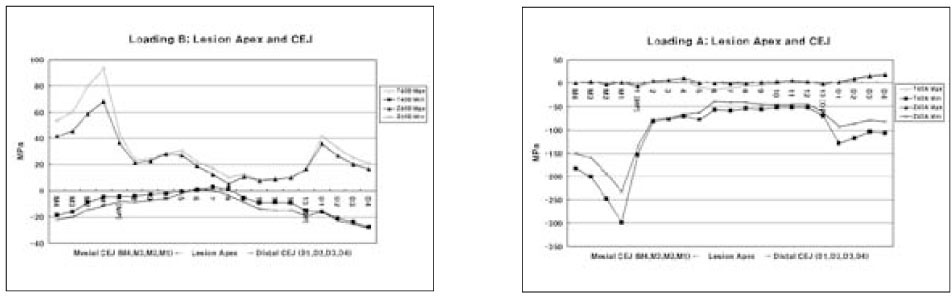 Figure 6
Figure 6 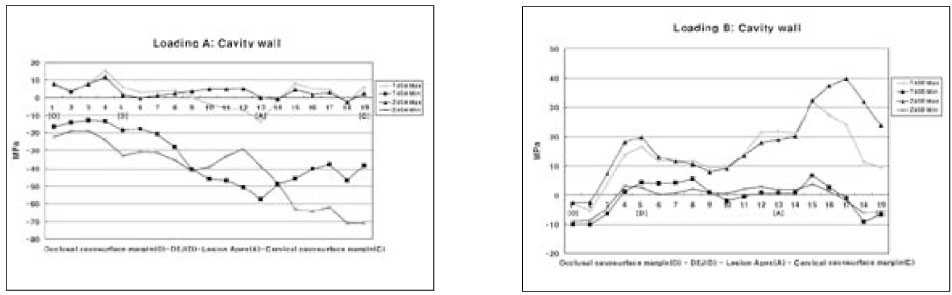 Figure 7
Figure 7 *: Excessive stresses over the failure range
O: Occlusal cavosurface margin
D: DEJ of occlusal wall
A: Lesion apex
C: Cervical cavosurface margin
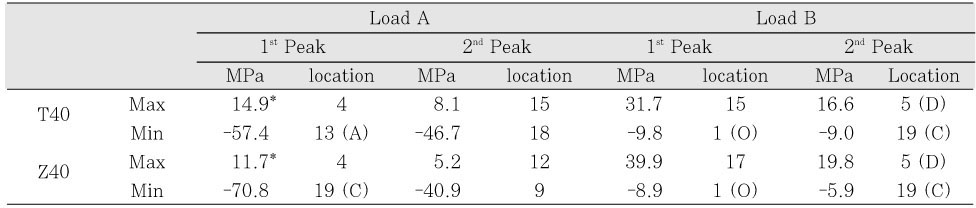 Download Table
Download Table IV. Discussion
V. Conclusion
- 1. Levitch LC, Bader JD, Shugars DA, Heymann HO. Non-carious cervical lesion. J Dent. 1994;22: 195-207.PubMed
- 2. Grippo JO, Simring M, Schreiner S. Attrition, abrasion, corrosion and abfration revisited - A new perspective on tooth surface lesions. J Am Dent Assoc. 2004;135: 1109-1118.PubMed
- 3. Grippo JO. Abfractions: A new classification of hard tissue lesions of tooth. J Esthet Dent. 1991;3(1):14-19.PubMed
- 4. Rees JS. A review of the Biomechanics of abfraction. Eur J Prosthodont Restor Dent. 2000;8(4):139-144.PubMed
- 5. Aw TC, Lepe X, Johnson GH, Mancl L. Characteristics of non-carious cervical lesion. J Am Dent Assoc. 2002;133: 725-733.PubMed
- 6. Bader JD, Levich LC, Shugars DA, Heymann HO, Mcclure F. How dentists classified & treated non-carious cervical lesions. J Am Dent Assoc. 1993;124: 46-54.PubMed
- 7. Grippo JO. Non-carious cervical lesions: The decision to ignore or restore. J Esthet Dent. 1992;4: 55-64.ArticlePubMed
- 8. Blunck U. Improving cervical restorations: A Review of Materials and Techniques. J Adhes Dent. 2001;3: 33-44.PubMed
- 9. Kemp-Scholte CM, Davidson CL. Complete marginal seal of class V resin composite restorations effected by increased flexibility. J Dent Res. 1990;69: 1240-1243.ArticlePubMedPDF
- 10. Kemp-Scholte CM, Davidson CL. Marginal integrity related to bond strength and strain capacity of composite resin restorative system. J Prosthet Dent. 1990;64: 658-664.PubMed
- 11. Van Meerbeek B, Peumans M, Gladys S, Braem M, Lambrechts P, Vanherle G. Three-year clinical effectiveness of four total-etch dentinal adhesive systems in cervical lesions. Quintessence Int. 1996;27: 775-784.PubMed
- 12. Ausiello P, Apicella A, Davidson CL. Effect of adhesive layer properties on stress distribution in composite restorations-a 3D finite element analysis. Dent Mater. 2002;18: 295-303.ArticlePubMed
- 13. Powell LV, Johnson GH, Gordon GE. Factors associated with clinical success of cervical abrasion/erosion restorations. Oper Dent. 1995;20: 7-13.PubMed
- 14. Litonjua LA, Andreana S, Patra AK, Cohen RE. An assessment of stress analyses in the theory of abfraction. Biomed Mater Eng. 2004;14: 311-321.PubMed
- 15. Rees JS. The role of cuspal flexure in the development of abfraction lesions: a finite element study. Eur J Oral Sci. 1998;106: 1028-1032.ArticlePubMedPDF
- 16. Tanaka M, Naito T, Yokota M, Kohno M. Finite element analysis of the possible mechanism of cervical lesion formation by occlusal force. J Oral Rehabil. 2003;30: 60-67.ArticlePubMedPDF
- 17. Rees JS, Hammadeh M. Undermining of enamel as a mechanism of abfraction lesion formation: a finite element study. Eur J Oral Sci. 2004;112: 347-352.PubMed
- 18. Son YH, Cho BH, Um CM. Finite element stress analysis of class V composite resin restoration subjected to cavity forms and placement methods. J Korean Acad Conserv Dent. 2000;25(1):91-108.
- 19. Um CM, Kwon HC, Son HH, Cho BH, Rim YI. Finite element analysis of stress distribution according to cavity design of class V composite resin filling. J Korean Acad Conserv Dent. 1999;24(1):67-74.
- 20. Lindehe J, Karring T. Textbook of Clinical Periodontology. 1989;2nd edition. Copenhagen: Munksgaard; 19-69.
- 21. Schroeder HE, Page RC. Periodontal Diseases. 1990;2nd edition. Philadelphia: Lea & Fabiger; 3-52.
- 22. Rubin C, Krishnamurthy N, Capilouto E, Yi H. Stress analysis of the human tooth using a three-dimensional finite element model. J Dent Res. 1983;62: 82-86.ArticlePubMedPDF
- 23. Katona TR, Winkler MM. Stress analysis of a bulk-filled class V light-cured composite restoration. J Dent Res. 1994;73(8):1470-1477.PubMed
- 24. Geramy A, Sharafoddin F. Abfraction: 3D analysis by means of the finite element method. Quintessence Int. 2003;34(7):526-533.PubMed
- 25. Le SY, Chiang HC, Huang HM, Shih YH, Chen HC, Dong DR, Lin CT. Thermo-debonding mechanisms in dentin bonding systems using finite element analysis. Biomaterials. 2001;22(2):113-123.ArticlePubMed
- 26. Kleverlaan CJ, Feilzer AJ. Polymerization shrinkage and contraction stress of dental resin composites. Dent Mater. 2005;article in press.ArticlePMC
- 27. Osborne-Smith KL, Burke FJT, Mc Farlane T, Wilson NHF. Effect of restored and unrestored non-carious cervical lesions on the fracture resistance of previously restored maxillary premolar teeth. J Dent. 1998;26: 427-433.PubMed
- 28. Kuroe T, Itoh H, Caputo AA, Konuma M. Biomechanics of cervical tooth structure lesions and their restoration. Quintessence Int. 2000;31(4):267-274.PubMed
- 29. Leinfelder KF. Restoration of abfracted lesions. Compendium. 1994;15: 1396-1400.PubMed
- 30. Yaman SD, Sahin M, Aydin C. Finite element analysis of strength characteristics of various resin based restorative material in Class V cavities. J Oral Rehabil. 2003;30: 630-641.PubMed
- 31. Browning WD, Bracket WW, Gilpatrick RO. Retention of microfilled and hybrid resin-based composite in noncarious class 5 lesions: A double-blind, randomized clinical trial. Oper Dent. 1999;24: 26-30.PubMed
- 32. Grippo JO, Simring M. Dental "erosion" revisited. J Am Dent Assoc. 1995;126: 619-630.ArticlePubMed
- 33. Lee WC, Eakle WS. Stress-induced cervical lesions: Review of advances in the past 10 years. J Prosthet Dent. 1996;75: 487-494.ArticlePubMed
REFERENCES
Tables & Figures
 Figure 3
Figure 3  Figure 2
Figure 2
 Figure 4
Figure 4 *: Excessive stresses over the failure range
A: Lesion apex, C: Cervical cavosurface margin, MP: Mesial point angle, DP: Distal point angle, NS: not significant
 Download Table
Download Table  Figure 5
Figure 5  Figure 6
Figure 6  Figure 7
Figure 7 *: Excessive stresses over the failure range
O: Occlusal cavosurface margin
D: DEJ of occlusal wall
A: Lesion apex
C: Cervical cavosurface margin
 Download Table
Download Table REFERENCES
- 1. Levitch LC, Bader JD, Shugars DA, Heymann HO. Non-carious cervical lesion. J Dent. 1994;22: 195-207.PubMed
- 2. Grippo JO, Simring M, Schreiner S. Attrition, abrasion, corrosion and abfration revisited - A new perspective on tooth surface lesions. J Am Dent Assoc. 2004;135: 1109-1118.PubMed
- 3. Grippo JO. Abfractions: A new classification of hard tissue lesions of tooth. J Esthet Dent. 1991;3(1):14-19.PubMed
- 4. Rees JS. A review of the Biomechanics of abfraction. Eur J Prosthodont Restor Dent. 2000;8(4):139-144.PubMed
- 5. Aw TC, Lepe X, Johnson GH, Mancl L. Characteristics of non-carious cervical lesion. J Am Dent Assoc. 2002;133: 725-733.PubMed
- 6. Bader JD, Levich LC, Shugars DA, Heymann HO, Mcclure F. How dentists classified & treated non-carious cervical lesions. J Am Dent Assoc. 1993;124: 46-54.PubMed
- 7. Grippo JO. Non-carious cervical lesions: The decision to ignore or restore. J Esthet Dent. 1992;4: 55-64.ArticlePubMed
- 8. Blunck U. Improving cervical restorations: A Review of Materials and Techniques. J Adhes Dent. 2001;3: 33-44.PubMed
- 9. Kemp-Scholte CM, Davidson CL. Complete marginal seal of class V resin composite restorations effected by increased flexibility. J Dent Res. 1990;69: 1240-1243.ArticlePubMedPDF
- 10. Kemp-Scholte CM, Davidson CL. Marginal integrity related to bond strength and strain capacity of composite resin restorative system. J Prosthet Dent. 1990;64: 658-664.PubMed
- 11. Van Meerbeek B, Peumans M, Gladys S, Braem M, Lambrechts P, Vanherle G. Three-year clinical effectiveness of four total-etch dentinal adhesive systems in cervical lesions. Quintessence Int. 1996;27: 775-784.PubMed
- 12. Ausiello P, Apicella A, Davidson CL. Effect of adhesive layer properties on stress distribution in composite restorations-a 3D finite element analysis. Dent Mater. 2002;18: 295-303.ArticlePubMed
- 13. Powell LV, Johnson GH, Gordon GE. Factors associated with clinical success of cervical abrasion/erosion restorations. Oper Dent. 1995;20: 7-13.PubMed
- 14. Litonjua LA, Andreana S, Patra AK, Cohen RE. An assessment of stress analyses in the theory of abfraction. Biomed Mater Eng. 2004;14: 311-321.PubMed
- 15. Rees JS. The role of cuspal flexure in the development of abfraction lesions: a finite element study. Eur J Oral Sci. 1998;106: 1028-1032.ArticlePubMedPDF
- 16. Tanaka M, Naito T, Yokota M, Kohno M. Finite element analysis of the possible mechanism of cervical lesion formation by occlusal force. J Oral Rehabil. 2003;30: 60-67.ArticlePubMedPDF
- 17. Rees JS, Hammadeh M. Undermining of enamel as a mechanism of abfraction lesion formation: a finite element study. Eur J Oral Sci. 2004;112: 347-352.PubMed
- 18. Son YH, Cho BH, Um CM. Finite element stress analysis of class V composite resin restoration subjected to cavity forms and placement methods. J Korean Acad Conserv Dent. 2000;25(1):91-108.
- 19. Um CM, Kwon HC, Son HH, Cho BH, Rim YI. Finite element analysis of stress distribution according to cavity design of class V composite resin filling. J Korean Acad Conserv Dent. 1999;24(1):67-74.
- 20. Lindehe J, Karring T. Textbook of Clinical Periodontology. 1989;2nd edition. Copenhagen: Munksgaard; 19-69.
- 21. Schroeder HE, Page RC. Periodontal Diseases. 1990;2nd edition. Philadelphia: Lea & Fabiger; 3-52.
- 22. Rubin C, Krishnamurthy N, Capilouto E, Yi H. Stress analysis of the human tooth using a three-dimensional finite element model. J Dent Res. 1983;62: 82-86.ArticlePubMedPDF
- 23. Katona TR, Winkler MM. Stress analysis of a bulk-filled class V light-cured composite restoration. J Dent Res. 1994;73(8):1470-1477.PubMed
- 24. Geramy A, Sharafoddin F. Abfraction: 3D analysis by means of the finite element method. Quintessence Int. 2003;34(7):526-533.PubMed
- 25. Le SY, Chiang HC, Huang HM, Shih YH, Chen HC, Dong DR, Lin CT. Thermo-debonding mechanisms in dentin bonding systems using finite element analysis. Biomaterials. 2001;22(2):113-123.ArticlePubMed
- 26. Kleverlaan CJ, Feilzer AJ. Polymerization shrinkage and contraction stress of dental resin composites. Dent Mater. 2005;article in press.ArticlePMC
- 27. Osborne-Smith KL, Burke FJT, Mc Farlane T, Wilson NHF. Effect of restored and unrestored non-carious cervical lesions on the fracture resistance of previously restored maxillary premolar teeth. J Dent. 1998;26: 427-433.PubMed
- 28. Kuroe T, Itoh H, Caputo AA, Konuma M. Biomechanics of cervical tooth structure lesions and their restoration. Quintessence Int. 2000;31(4):267-274.PubMed
- 29. Leinfelder KF. Restoration of abfracted lesions. Compendium. 1994;15: 1396-1400.PubMed
- 30. Yaman SD, Sahin M, Aydin C. Finite element analysis of strength characteristics of various resin based restorative material in Class V cavities. J Oral Rehabil. 2003;30: 630-641.PubMed
- 31. Browning WD, Bracket WW, Gilpatrick RO. Retention of microfilled and hybrid resin-based composite in noncarious class 5 lesions: A double-blind, randomized clinical trial. Oper Dent. 1999;24: 26-30.PubMed
- 32. Grippo JO, Simring M. Dental "erosion" revisited. J Am Dent Assoc. 1995;126: 619-630.ArticlePubMed
- 33. Lee WC, Eakle WS. Stress-induced cervical lesions: Review of advances in the past 10 years. J Prosthet Dent. 1996;75: 487-494.ArticlePubMed
Citations

- The influence of occlusal loads on stress distribution of cervical composite resin restorations: A three-dimensional finite element study
Chan-Seok Park, Bock Hur, Hyeon-Cheol Kim, Kwang-Hoon Kim, Kwon Son, Jeong-Kil Park
Journal of Korean Academy of Conservative Dentistry.2008; 33(3): 246. CrossRef

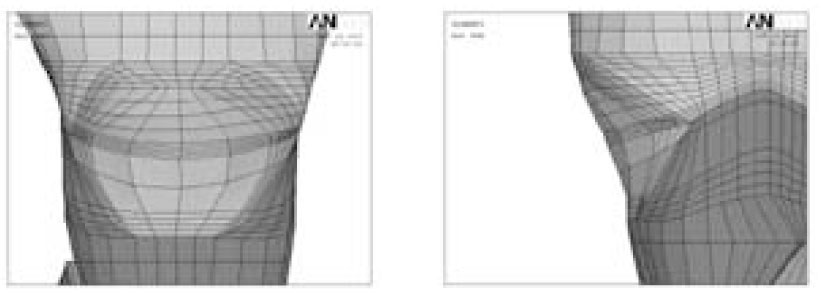

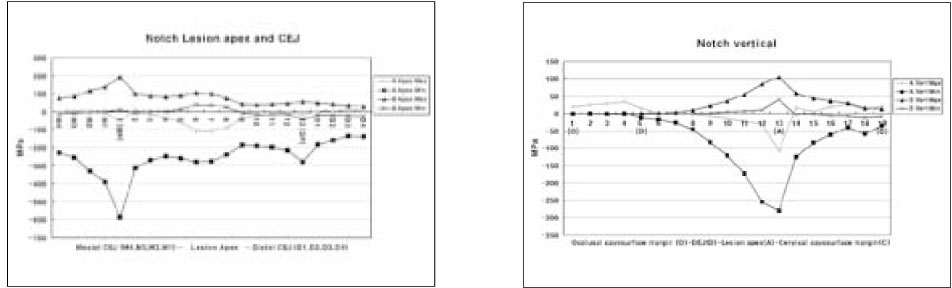
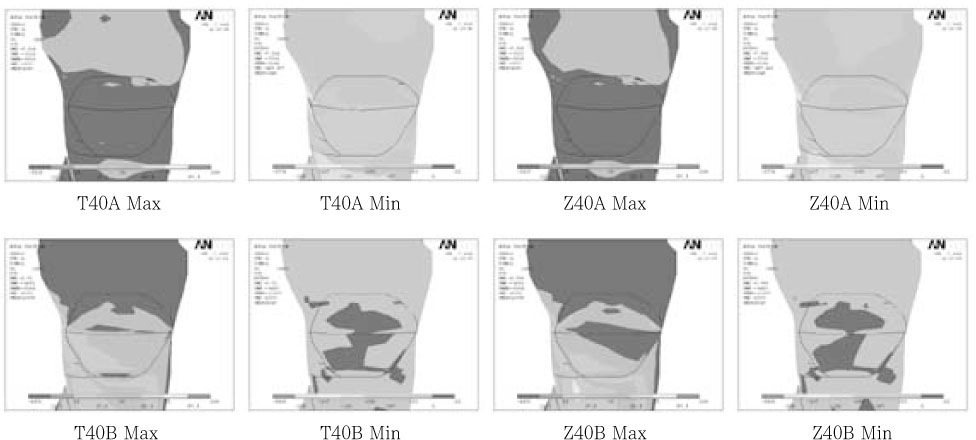
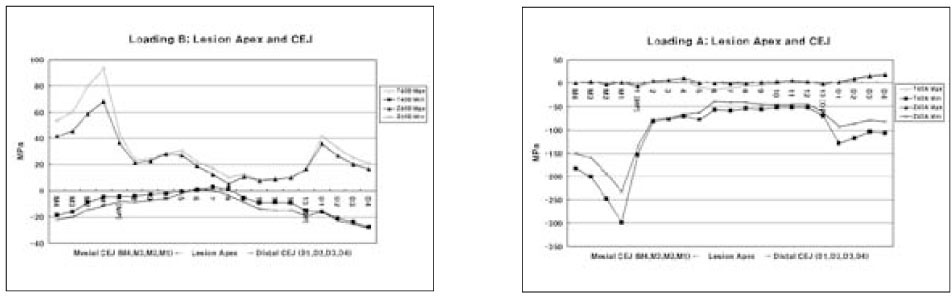
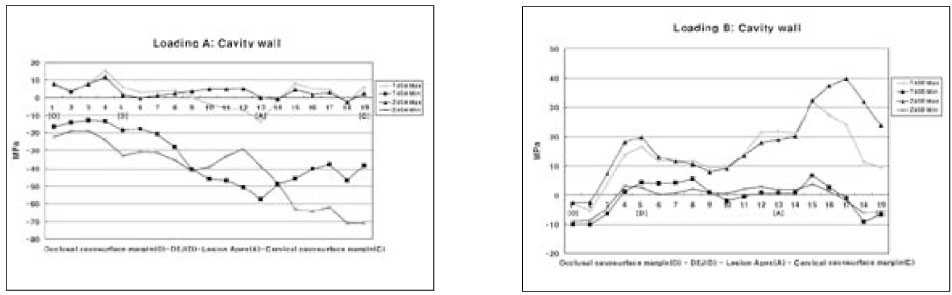
Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6
Figure 7
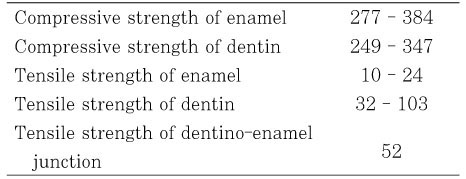
Mechanical properties of the tooth used in the study
Mechanical properties of the materials used in the study
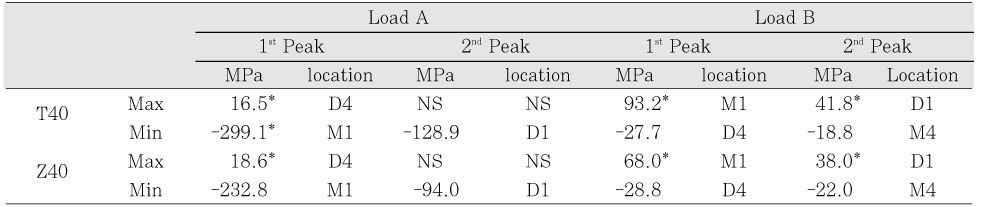
Mechanical properties of teeth (MPa)
*: Litonjua et al.14)
Peak stresses of each area before restoration
*: Excessive stresses over the failure range
A: Lesion apex, C: Cervical cavosurface margin, MP: Mesial point angle, DP: Distal point angle, NS: not significant
Peak stresses of lesion apex and CEJ
*: Excessive stresses over the failure range
Peak stresses of vertical cavity wall
*: Excessive stresses over the failure range
O: Occlusal cavosurface margin
D: DEJ of occlusal wall
A: Lesion apex
C: Cervical cavosurface margin
a: Katona et al.
a: Katona et al.
*: Litonjua et al.
*: Excessive stresses over the failure range
A: Lesion apex, C: Cervical cavosurface margin, MP: Mesial point angle, DP: Distal point angle, NS: not significant
*: Excessive stresses over the failure range
*: Excessive stresses over the failure range
O: Occlusal cavosurface margin
D: DEJ of occlusal wall
A: Lesion apex
C: Cervical cavosurface margin

 KACD
KACD


 ePub Link
ePub Link Cite
Cite